
Ivanov, Yu. N. 2002. Mutagenesis and sex ratio in Drosophila melanogaster. Dros. Inf. Ser. 85: 96-105.
|
|
|
|||
|
PDF
file
|
|
|||
Mutagenesis and
sex ratio in Drosophila melanogaster.
Ivanov, Yu. N. Institute of Cytology and Genetics, Siberian Division of Russian Academy of Sciences, Novosibirsk, 630090, Russia. FAX: (3832)33 12 78. E-mail: iyn@bionet.nsc.ru.
According to
Catcheside and Lea (1945), already the first researchers of mutagenesis (Muller,
1928, 1940; Hanson, 1928;
Gowen and Gay, 1933; Bauer, 1939; Catcheside and Lea, 1945) found that when males of D. melanogaster
were irradiated, in their
![]() progeny a reduction of sex ratio
progeny a reduction of sex ratio
![]() was observed, i.e. the numerical female to male ratio decreased as compared
to non-irradiated control. (Here and below,
was observed, i.e. the numerical female to male ratio decreased as compared
to non-irradiated control. (Here and below,
![]() stands for the 1st
generation, and
stands for the 1st
generation, and
![]() for its parents.) Catcheside
and Lea summarized the data of all authors and found that the dependence of
the sex ratio on the dose of irradiation of
for its parents.) Catcheside
and Lea summarized the data of all authors and found that the dependence of
the sex ratio on the dose of irradiation of
![]() males in Roentgens was a decreasing
function
males in Roentgens was a decreasing
function
![]() , where
, where
![]() is the sex ratio in the control.
If one assumes that the function
is the sex ratio in the control.
If one assumes that the function
![]() is fit for all possible doses,
then it seems that increasing the dose one can decrease the sex ratio practically
to zero.
is fit for all possible doses,
then it seems that increasing the dose one can decrease the sex ratio practically
to zero.
A usual consequence
of mutagenesis is formation of dominant lethal mutations (DLM), which reduce the number of
![]() offspring of mutagen-treated
parents; therefore, it is natural to attribute the reduction of the sex ratio
in mutagenesis to the effect of DLM that is selective with respect to sex
(Catcheside and Lea, 1945; Hadorn,
1961; Ivanov, 1998a, b). According
to the common notion, DLM are deletions of euchromatin (McClintock, 1941;
Hadorn, 1961) and its point damages (usually genic mutations) (Ivanov,
1998b) with immediate lethal effect in the very first zygote. If the lethal
effect of the mutation is not manifested in the first zygote, which it entered
after its formation, such mutation is not recorded as a DLM, even if it turns
out to be lethal in subsequent generations. The mutation is called dominant
lethal only because it displays a lethal effect in heterozygote, although
it would have turned out to be an ordinary recessive lethal or visible mutation
had it not been eliminated immediately as DLM.
offspring of mutagen-treated
parents; therefore, it is natural to attribute the reduction of the sex ratio
in mutagenesis to the effect of DLM that is selective with respect to sex
(Catcheside and Lea, 1945; Hadorn,
1961; Ivanov, 1998a, b). According
to the common notion, DLM are deletions of euchromatin (McClintock, 1941;
Hadorn, 1961) and its point damages (usually genic mutations) (Ivanov,
1998b) with immediate lethal effect in the very first zygote. If the lethal
effect of the mutation is not manifested in the first zygote, which it entered
after its formation, such mutation is not recorded as a DLM, even if it turns
out to be lethal in subsequent generations. The mutation is called dominant
lethal only because it displays a lethal effect in heterozygote, although
it would have turned out to be an ordinary recessive lethal or visible mutation
had it not been eliminated immediately as DLM.
The theory explains
the described shift of the sex ratio as follows. Due to the fact that the
changes induced in the euchromatin X chromosome of X spermia make a greater
contribution to DLM than the changes induced in the heterochromatin Y chromosome
of Y spermia do, a mutagen-treated male transmits the obtained injuries more
often to his daughters XX than to
his sons XY. (Bold letters
here designate chromosomes brought into the zygote by the male, i.e.
mutagen-treated ones.) That is why in
![]() females die more frequently than
males and sex ratio decreases. The higher the mutagen dose, the more DLM arise,
and the more the sex ratio must decrease, so that it could serve as a measure
for the number of DLM.
females die more frequently than
males and sex ratio decreases. The higher the mutagen dose, the more DLM arise,
and the more the sex ratio must decrease, so that it could serve as a measure
for the number of DLM.
Using these assumptions,
the author has worked out a schedule of experiments for estimating the average
number of DLM in the genome of D. melanogaster (Ivanov, 1998a, b) in order to confirm and specify the
estimate of the number of DLM obtained earlier by a more direct method –
by zygote survival (Ivanov, 1998c). Already the first three experiments gave
not quite satisfactory results, since the treatment of
![]() males with ethylmethane sulphonate
(EMS) (equivalent by its mutability in the X chromosome to a
19350 R g-irradiation) and with g-irradiation
in the doses of 1500 and 2500 R gave
males with ethylmethane sulphonate
(EMS) (equivalent by its mutability in the X chromosome to a
19350 R g-irradiation) and with g-irradiation
in the doses of 1500 and 2500 R gave
smaller deviations from the normal sex ratio than it was expected, so that the estimates of the average DLM number per genome turned out to be understated. Wanting to obtain
more reliable results, the author replicated the experiment twice at higher g-ray doses, using in one case (strain Oregon-R) irradiation of females as control.
The results were unexpected and discouraging. They were so puzzling that doubts arose concerning the correctness of dosage, which, however, turned out to be absolutely unwarranted. Firstly, despite the dose of 7000 R, the sex ratio did not change. Secondly, it was expected that irradiation of females would cause an increase of the sex ratio, since all the recessive lethals induced in female’s X chromosomes, having got into hemizygote in her sons, had to behave as DLM and reduced the number of males. However, there were no symptoms thereof.
Studies of the
effect of g-irradiation
of
![]() males and females on the sex
ratio were specially continued on a rather large number of cultures and flies
in the cultures and at considerable irradiation doses (up to 15000 R), but
without any effect predicted by the theory. All the author’s data on
sex ratio in
males and females on the sex
ratio were specially continued on a rather large number of cultures and flies
in the cultures and at considerable irradiation doses (up to 15000 R), but
without any effect predicted by the theory. All the author’s data on
sex ratio in
![]() under the influence of mutagens
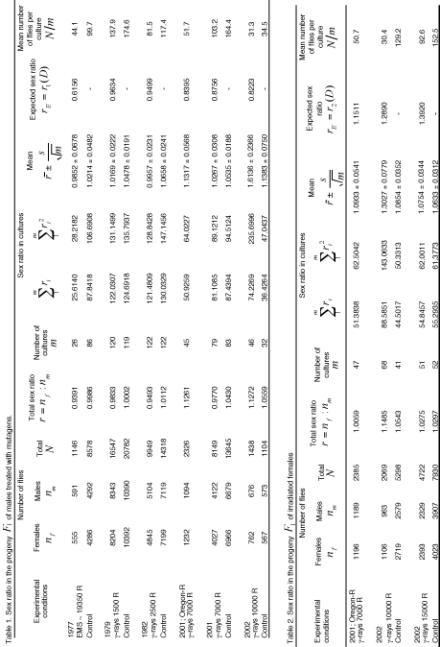
on males are presented in Table 1, and on females, in Table 2.
under the influence of mutagens
on males are presented in Table 1, and on females, in Table 2.
In the two tables,
the column “Experimental conditions” indicates the year, fly strain
and irradiation dose: no indication means the strain Canton-S, but the use
of Oregon-R is indicated. The control always represents replication of the
experiment presented in the line situated above, but without irradiation.
Columns with sums
![]() and
and
![]() are presented for the sake of
convenience of variance analysis.
are presented for the sake of
convenience of variance analysis.
The expected sex ratio in Table 1 and 2 was calculated differently. In irradiation of males (Table 1) it was calculated by Catcheside and Lea formula
![]() (1)
(1)
where
![]() is the total sex ratio in the
control. In the absence of ordinary control, it was assumed that
is the total sex ratio in the
control. In the absence of ordinary control, it was assumed that
![]() . In irradiation of females (Table 2), for
. In irradiation of females (Table 2), for
![]() it was necessary to use a different
formula deduced as follows.
it was necessary to use a different
formula deduced as follows.
Dependence of
the average number
![]() of recessive lethal mutations
(RLM) and visible mutations (VM)
induced in X chromosome of males on the irradiation dose
of recessive lethal mutations
(RLM) and visible mutations (VM)
induced in X chromosome of males on the irradiation dose
![]() in R is described by a linear
function
in R is described by a linear
function
![]() ,
(2)
,
(2)
which was obtained from all our
experiments on measurement of mutability in male’s X chromosome at various
doses of g-irradiation. (Its coefficients with errors are
![]() and
and
![]() .) Let us assume that dependence of mutability on the dose in ova is the same
as that in spermia. Since RLM arising in ova affect males like DLM do, because
they turn out to be in hemizygote, in order to take into account the death
of males due to irradiation of parental females, let us find the dependence
of the average number
.) Let us assume that dependence of mutability on the dose in ova is the same
as that in spermia. Since RLM arising in ova affect males like DLM do, because
they turn out to be in hemizygote, in order to take into account the death
of males due to irradiation of parental females, let us find the dependence
of the average number
![]() of RLM induced in the X chromosome
of ova on the irradiation dose
of RLM induced in the X chromosome
of ova on the irradiation dose
![]() . RLM make up
. RLM make up
![]() of the whole number of arising
RLM and VM, therefore the sought relation will be expressed by the formula
of the whole number of arising
RLM and VM, therefore the sought relation will be expressed by the formula
![]() .
(3)
.
(3)
Let us designate
in the equation (3) the free term, or the number of spontaneous RLM in X chromosome,
as
![]() , and the coefficient as
, and the coefficient as
![]() . The probability of survival of a male produced by non-irradiated parents
is
. The probability of survival of a male produced by non-irradiated parents
is
![]() , where
, where
![]() is the frequency of arising of
spontaneous RLM in X chromosome. The probability of survival of a male produced
by female irradiated with a dose
is the frequency of arising of
spontaneous RLM in X chromosome. The probability of survival of a male produced
by female irradiated with a dose
![]() is
is
![]() , where
, where
![]() is the frequency of arising of
RLM in X chromosome induced by the dose
is the frequency of arising of
RLM in X chromosome induced by the dose
![]() . Let the sex ratio without irradiation be equal to unit,
. Let the sex ratio without irradiation be equal to unit,
![]() and
and
![]() be the proportions of females
and males in the progeny of females irradiated with the dose
be the proportions of females
and males in the progeny of females irradiated with the dose
![]() , respectively, and
, respectively, and
![]() be the multiplicity of decrease
of the proportion of flies of each sex due to mutations (autosomal etc.) equally
affecting the mortality of both sexes, at the dose
be the multiplicity of decrease
of the proportion of flies of each sex due to mutations (autosomal etc.) equally
affecting the mortality of both sexes, at the dose
![]() . Then the expected sex ratio in irradiation of female with the dose
. Then the expected sex ratio in irradiation of female with the dose
![]() is
is
![]() .
.
The multiplier
![]() in the numerator is necessary
to make the sex ratio without irradiation equal to 1. Substituting the numerical
value
in the numerator is necessary
to make the sex ratio without irradiation equal to 1. Substituting the numerical
value
![]() into the obtained expression
and introducing the multiplier
into the obtained expression
and introducing the multiplier
![]() , which corrects the result for the total sex ratio in the control, we obtain
definitely:
, which corrects the result for the total sex ratio in the control, we obtain
definitely:
![]() .
(4)
.
(4)
It is by this formula that theoretically expected sex ratio in Table 2 was calculated.
Now one can see that the real sex ratio in mutagenesis is different from the expected one, most often conserving value close to unit, i.e. it does not obey the tendency predicted by the theory. If the reader finds that the assumptions under which formula (4) for the case of irradiated females was obtained are not quite correct, let him compare the real sex ratio with irradiation of females not with the theoretically expected one, but with the control. The equal sex ratios in the experiment and in the control also demonstrate inconsistency of the theory according to which it must change due to mutagenesis.
The use of high
doses of mutagens usually causes a considerable mortality of flies due to
induction of DLM, the population density in cultures decreasing extremely,
which probably could tell on the sex ratio. For this reason, for compensation
of flies’ death from DLM and for at least a relative leveling of population
density in cultures in the experiment and in the control, at high irradiation
doses we put into cultures many parental females – often over 20 –
instead of the usual 1 – 2 in control cultures. The columns of tables
entitled “Mean number of flies in culture
![]() ” show how useful this turned out to be. Thanks to this, the
total number of flies in experiments increased considerably. The first researchers
did not resort to such measures, and, probably, it is because of this that
they obtained results different from ours. So, Catcheside and Lea (1945) obtained
their sex ratio of
” show how useful this turned out to be. Thanks to this, the
total number of flies in experiments increased considerably. The first researchers
did not resort to such measures, and, probably, it is because of this that
they obtained results different from ours. So, Catcheside and Lea (1945) obtained
their sex ratio of
![]() for the highest dose of
11420 R on only 13 flies.
for the highest dose of
11420 R on only 13 flies.
Let us first consider the experiments with mutagenic treatment of males (Table 1).
Chi-square test
shows a statistically significant heterogeneity of the total sex ratio
![]() in the total set of all the experimental
and control samples (
in the total set of all the experimental
and control samples (
![]() at
at
![]() ,
,
![]() ). The same test shows a statistically significant heterogeneity of the set
of experimental samples (
). The same test shows a statistically significant heterogeneity of the set
of experimental samples (
![]() at
at
![]() ,
,
![]() ) and homogeneity of control samples (
) and homogeneity of control samples (
![]() at
at
![]() ), wherefrom it follows that the heterogeneity of the total sex ratio is caused
by the mutagens, but not by any other factor.
), wherefrom it follows that the heterogeneity of the total sex ratio is caused
by the mutagens, but not by any other factor.
Variance analysis
leads to the same conclusions for the average sex ratio in cultures. The hypothesis
of equality of mean values for sex ratio in all the experimental samples is
discarded as incredible at the significant level of
![]() , but the same hypothesis for control samples is not rejected. In two-way
analysis, no influence of the general experimental conditions on the sex ratio
is found, and the influence of mutagenic treatment of males is rather significant
(
, but the same hypothesis for control samples is not rejected. In two-way
analysis, no influence of the general experimental conditions on the sex ratio
is found, and the influence of mutagenic treatment of males is rather significant
(
![]() ). For the sake of brevity, variance analysis is not presented, but it is
easy to reconstruct it since Table 1 and 2 contain all necessary data.
). For the sake of brevity, variance analysis is not presented, but it is
easy to reconstruct it since Table 1 and 2 contain all necessary data.
Although the influence of mutagenesis on sex ratio is statistically significant, it does not coincide with the theoretical one. Thus, in some cases although the shift of the sex ratio in the experiment in direction of decrease versus the control coincides with the theoretical expectation, it is only with respect to direction. In fact it is, as a rule, insignificant and much weaker than expected on the basis of the size of X chromosome whose contribution to the genome of D. melanogaster makes up about 19%.
The mean sex
ratio in culture in experimental samples was 1.085 ±
0.029 (0.985 – 1.614), in control ones it was 1.058 ±
0.013 (1.021 – 1.138), and in all samples 1.071 ±
0.015. Regression analysis of the dependence of the mean sex ratio in cultures
on the irradiation dose for males gives a value of regression coefficient
![]() . The value for Student test
. The value for Student test
![]() is equal to
is equal to
![]() , so that the hypothesis that the coefficient of regression is zero
is not rejected. The average sex ratio at all the doses remains the same.
Since the treatment of males with mutagens does not cause any decrease of
sex ratio even at high doses, and yet the influence of mutagens on the sex
ratio is statistically significant, we face here a case when there is dependence
but no correlation. So what does this dependence consist in?
, so that the hypothesis that the coefficient of regression is zero
is not rejected. The average sex ratio at all the doses remains the same.
Since the treatment of males with mutagens does not cause any decrease of
sex ratio even at high doses, and yet the influence of mutagens on the sex
ratio is statistically significant, we face here a case when there is dependence
but no correlation. So what does this dependence consist in?
At first it seemed
that mutagenesis increased the variability of sex ratio across cultures of
the sample, but this is not the case. Coefficient of correlation between the
irradiation dose and characteristics of sex ratio variability in cultures
are
![]() for standard deviation
for standard deviation
![]() , and
, and
![]() for coefficient of variation
for coefficient of variation
![]() at 10 degrees of freedom.
The hypothesis of absence of correlation is not rejected. Therefore, the variability
of sex ratio does not correlate with the dose, the average value does not
correlate, either. Irradiation does not increase the sex ratio variability
in the sample, but simply makes the distribution of sex ratio on the whole
unstable, influencing irregularly all its characteristics.
at 10 degrees of freedom.
The hypothesis of absence of correlation is not rejected. Therefore, the variability
of sex ratio does not correlate with the dose, the average value does not
correlate, either. Irradiation does not increase the sex ratio variability
in the sample, but simply makes the distribution of sex ratio on the whole
unstable, influencing irregularly all its characteristics.
We see that the formula of Catcheside and Lea does not describe the dependence under discussion. Our experiments cover a time stretch of about quarter of a century, they were made under various conditions, but, as variance analysis demonstrated, this has not affected the sex ratio. The absence of influence of other conditions than mutagenic treatment of males shows that equal results of the first researchers were not at all caused by similarity of external conditions under which they performed their observations. Similarity of conditions might consist in the fact that at the beginning, when the fly cultivation technique was just starting to be spread, equal procedures, strains, media, temperatures and other factors could be used, which could result in equal effects on the sex ratio. Why did the first researchers observe an equal direction of shift of the sex ratio, remains an enigma. Could it not be a result of prejudice unconsciously widespread in a mysterious way? Is it not surprising that X and Y spermia seem to bring into the zygote an equal number of induced DLM, despite the large difference in their genetic contents, and could one have supposed this a priori? One can hardly doubt, however, as to our experimental data, which seem to meet all the requirements of reliability. Science knows the existence of latent, subconscious and unpremeditated tendentiousness (Taylor, 1987) when a researcher becomes like a seller who has been noticed, when settling accounts, to be mistaken in his own favor. It cannot be ruled out it is just this that has determined the slight shift of sex ratio in our first experiments of 1977, 1979 and 1982 on estimation of the number of DLM (Ivanov, 1998a, b).
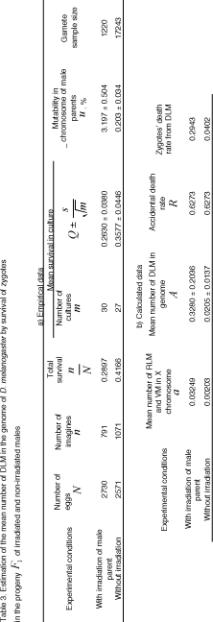
In connection with the phenomenon of equal mortality of sexes in mutagenesis, it became necessary to revise the earlier obtained results associated with the estimation of the number of DLM by the sex ratio, and the method (formulae) of their calculation by zygotes’ survival (Ivanov, 1998a, b, c). The error consisted in the fact that, ignoring large errors of the results obtained by the sex ratio, we added and averaged all the data on the number of DLM in order to minimize the total error. It is only thanks to the fact that the averaging was performed with weights inverse to square errors, that the total result did not deviate much from the more correct experimental value. Now that it has become clear that we ought not to pool the results, we have discarded the unreliable data obtained from the sex ratio and used for estimation of the number of DLM by the survival of zygotes the results of an additional experiment discarded earlier because of the considerable chance mortality. Since in the zygote survival method the chance mortality, which is equal in the control and in the experiment, is ruled out, its value does not influence the result. The additional experiment was not analyzed at its time and was ignored for a long time as a failure. The data of this experiment on estimation of the average number of DLM in the genome of D. melanogaster, both spontaneous and induced with the dose of 1500 R, are presented in Table 3. The theory and technique of this experiment are described in our earlier work (Ivanov, 1998c).
Now the calculations
have been simplified. In them, only one, average, or intermediate, genome
figures, because the necessity of considering two genomes – complete
(with X chromosome) and incomplete (with Y chromosome) – has disappeared:
both of them bring into the zygote on the average equal numbers of DLM, which
follows from all our experiments on the influence of mutagenesis on the sex
ratio. The formula for the mean number
![]() of spontaneous DLM in the genome
now is as follows
of spontaneous DLM in the genome
now is as follows
 ,
(5)
,
(5)
where
![]() and
and
![]() are the mean survival of zygotes
in culture in the experiment and in the control, respectively, and
are the mean survival of zygotes
in culture in the experiment and in the control, respectively, and
![]() and
and
![]() are mutability in males’
X chromosome in the experiment and in the control, respectively. The mean
number
are mutability in males’
X chromosome in the experiment and in the control, respectively. The mean
number
![]() of induced DLM in the genome
is
of induced DLM in the genome
is
 .
(6)
.
(6)
The results in Table 3b have been calculated by formulae (5) and (6), however, one can specify them.
Given the dependence of the average number of mutations induced in
males’ X chromosome on the irradiation dose as linear function (2),
we shall find the dependence of multiplicity of increase of the number of
mutations over the spontaneous level
![]() on the irradiation dose as a
linear function
on the irradiation dose as a
linear function
![]() .
(7)
.
(7)
(The estimate of dispersion of
multiplicity
![]() at the dose
at the dose
![]() is
is
![]() .) Then the average number of spontaneous DLM in the genome is
.) Then the average number of spontaneous DLM in the genome is
![]() ,
(8)
,
(8)
and the mean number of DLM induced
in the genome after irradiation of males is
![]() .
.
The errors of
calculations by formula (8) are estimated more simply and correctly, so we
recalculated by it the average number of spontaneous and induced DLM in the
genome in both experiments. At the dose of 1500 R which was used, the multiplicity
of increase of the number of mutations is
![]() ; the mean number of spontaneous DLM in the genome is
; the mean number of spontaneous DLM in the genome is
![]() in the experiment with
a low chance mortality
in the experiment with
a low chance mortality
![]() , and
, and
![]() in the additional experiment
with a high chance mortality
in the additional experiment
with a high chance mortality
![]() ; the mean number of DLM in the genome irradiated with 1500 R is
; the mean number of DLM in the genome irradiated with 1500 R is
![]() in the former experiment
and
in the former experiment
and
![]() in the latter. A weighted
averaging of respective data by formula
in the latter. A weighted
averaging of respective data by formula
![]() results in the following
estimates, which may be used for practical calculations.
results in the following
estimates, which may be used for practical calculations.
The weighted mean number of spontaneous DLM in the genome is
![]() .
(9)
.
(9)
The weighted mean number of DLM induced in the genome by the dose of 1500 R is
![]() .
(10)
.
(10)
From the data of (9) and (10), dependence of the mean number of DLM induced in the genome on the dose of irradiation of males is deduced as linear function
![]() ,
(11)
,
(11)
in which coefficients with errors
are
![]() and
and
![]() . Other researchers measured the number of DLM not as their mean number
in the genome, but estimated it directly by the death of zygotes (Catcheside,
Lea, 1945; Fano, Demerec, 1941). That is why they never observed the simple
linear dependence on the dose. From it, formulae for calculation of zygotes’
survival at various doses of irradiation of parent of one sex or of both sexes
together are deduced.
. Other researchers measured the number of DLM not as their mean number
in the genome, but estimated it directly by the death of zygotes (Catcheside,
Lea, 1945; Fano, Demerec, 1941). That is why they never observed the simple
linear dependence on the dose. From it, formulae for calculation of zygotes’
survival at various doses of irradiation of parent of one sex or of both sexes
together are deduced.
Let us assume
that irradiation of males and females makes the same DLM contribution to their
![]() progeny. Let us ignore the chance
death, i.e. assume it to be zero. If it becomes necessary for correction of
the survival value, it will to be easy to introduce it into our formulae as
a multiplier
progeny. Let us ignore the chance
death, i.e. assume it to be zero. If it becomes necessary for correction of
the survival value, it will to be easy to introduce it into our formulae as
a multiplier
![]() . The probability of survival is the probability of formation of a DLM-free
zygote. If, as it is assumed,
. The probability of survival is the probability of formation of a DLM-free
zygote. If, as it is assumed,
![]() and
and
![]() are mean DLM numbers in the genome
in spontaneous and induced mutagenesis, respectively, then, due to irradiation
of one parent with the dose
are mean DLM numbers in the genome
in spontaneous and induced mutagenesis, respectively, then, due to irradiation
of one parent with the dose
![]() the survival of zygotes is
the survival of zygotes is
![]() , and with irradiation of both parent
, and with irradiation of both parent
![]() . Substituting into these expressions the estimate (9) of the number
. Substituting into these expressions the estimate (9) of the number
![]() of spontaneous DLM, and instead
of
of spontaneous DLM, and instead
of
![]() its expression (11), we shall
obtain the dependence of survival of zygotes on the irradiation dose:
its expression (11), we shall
obtain the dependence of survival of zygotes on the irradiation dose:
![]() with irradiation of one
parent and
with irradiation of one
parent and
![]() with irradiation of both
parents.
with irradiation of both
parents.
So, when males are irradiated
with the dose of 15000 R, then
![]() , i.e. about
, i.e. about
![]() of
of
![]() zygotes survive (are free from
DLM); therefore, in order to obtain in culture the same number of flies as
without irradiation, one has to increase the number of parental females by
more than 20 times.
zygotes survive (are free from
DLM); therefore, in order to obtain in culture the same number of flies as
without irradiation, one has to increase the number of parental females by
more than 20 times.
The work “Factors of spontaneous mutation, mutability in large chromosomes, and mortality from dominant lethals in D. melanogaster” (Ivanov, 1999) also requires some corrections. The decomposition of the mutation process in the genome of D. melanogaster was practically not changed, but the formulae for calculation of zygotes’ mortality from DLM by mutability in the given chromosome became simplified and partially turned out to be superfluous due to similarity in survival of sexes. Let us deduce them again.
If
![]() is the frequency of occurrence
of DLM in the genome, then the mortality of zygotes from DLM, according to
the new statement, is equal in both sexes and equal to
is the frequency of occurrence
of DLM in the genome, then the mortality of zygotes from DLM, according to
the new statement, is equal in both sexes and equal to
![]() .
(12)
.
(12)
We shall find the
![]() value by the frequency
value by the frequency
![]() of arising of RLM and VM in the
of arising of RLM and VM in the
![]() -th chromosome and by its proportion
-th chromosome and by its proportion
![]() in the whole genome. Let
in the whole genome. Let
![]() be the arising frequency of RLM
and VM in the whole genome. We have
be the arising frequency of RLM
and VM in the whole genome. We have
![]() and, according to the
decomposition of the mutation process,
and, according to the
decomposition of the mutation process,
![]() . Then
. Then
![]() ,
,
![]() , and the sought mortality of zygotes (12) is
, and the sought mortality of zygotes (12) is
![]() ,
(13)
,
(13)
which coincides with the previous
formula of mortality for females. The shares of X chromosome and chromosome
2 in the genome are
![]() and
and
![]() , respectively. Substituting these numbers into formula (13), we obtain for
each chromosome the expression of the zygotes’ mortality on DLM as a
function of mutability in the given chromosome:
, respectively. Substituting these numbers into formula (13), we obtain for
each chromosome the expression of the zygotes’ mortality on DLM as a
function of mutability in the given chromosome:
![]() ;
(14)
;
(14)
![]() .
.
One can obtain an estimate of
the maximal mortality of zygotes from DLM in populations by means of substituting
into formulae (14) the highest rates of spontaneous mutability
![]() which are known for these chromosomes:
which are known for these chromosomes:
![]() and
and
![]() . The highest values for mortality from DLM are
. The highest values for mortality from DLM are
![]() and
and
![]() . Hence the spontaneous mutability completely caused by biotic factors
(Ivanov, 1998a, 1999) is one of important factors of mortality and of limitation
of the numbers of species.
. Hence the spontaneous mutability completely caused by biotic factors
(Ivanov, 1998a, 1999) is one of important factors of mortality and of limitation
of the numbers of species.
Finally, let
us consider the experiments on irradiation of
![]() females (Table 2). Their statistical
treatment has led to the following conclusions.
females (Table 2). Their statistical
treatment has led to the following conclusions.
The test for
homogeneity of
![]() for the total sex ratio in all
the 5 samples, experimental and control ones, shows that the homogeneity hypothesis
is not rejected:
for the total sex ratio in all
the 5 samples, experimental and control ones, shows that the homogeneity hypothesis
is not rejected:
![]() at
at
![]() . Experimental samples do not differ from control in the total sex ratio.
. Experimental samples do not differ from control in the total sex ratio.
Simple one-way
variance analysis for checking the equality of average values, and two-way
analysis where irradiation and general experimental conditions are the factors
demonstrate that irradiation does influence significantly the mean sex ratio
in sample cultures at the significant level of
![]() , whereas the general conditions do not.
, whereas the general conditions do not.
Like in irradiation
of males, the influence of irradiation of females on the distribution of sex
ratio across
![]() cultures consists in destabilization
of the mean sex ratio in absence of its shift in the direction of increase
with an increase of the dose, i.e. a shift expected theoretically.
cultures consists in destabilization
of the mean sex ratio in absence of its shift in the direction of increase
with an increase of the dose, i.e. a shift expected theoretically.
The mean sex
ratio in experimental samples was 1.174 ± 0.038 (1.075 –
1.303), in control samples it was 1.090 ± 0.026 (1.063 –
1.138), and over all the samples it was 1.138 ±
0.024. Regression analysis of dependence of the sex ratio on the dose of irradiation
of females gives the value for coefficient of regression
![]() . The value for Student test
. The value for Student test
![]() is
is
![]() , i.e. the coefficient of regression is not significantly different from zero,
and the mean sex ratio is practically independent of the dose.
, i.e. the coefficient of regression is not significantly different from zero,
and the mean sex ratio is practically independent of the dose.
The phenomenon
of weak dependence of sex ratio on the dose of irradiation of
![]() females with conservation of
a value close to unit or to the level without irradiation seem not to have
been noticed earlier, otherwise, on the strength of its paradoxical character,
it would have raised some problems. The absence of an elevated mortality of
males that originated from mutagen-treated ova is extremely puzzling.
females with conservation of
a value close to unit or to the level without irradiation seem not to have
been noticed earlier, otherwise, on the strength of its paradoxical character,
it would have raised some problems. The absence of an elevated mortality of
males that originated from mutagen-treated ova is extremely puzzling.
First of all, doubts arise as to whether irradiation has taken place. People who worked on the g-irradiator strongly protested against the hypothesis that the IGUR-1 device with caesium-137 that was used worked poorly, since the radiation supervisor regularly controls its power necessary for calculation of irradiation time by the dose, or the dose by the time. At considerable doses, irradiation effect must be well noticeable by the death of flies from DLM. Although we took measures that the number of flies in cultures were considerable and should not be different in the experiment and in the control, i.e. by many times increased the number of parental females in irradiation, yet, as one can judge by the data of the last columns in Tables 1 and 2, the average number of flies in experimental cultures was always smaller than that in the control, so that the effect of irradiation remained noticeable, and there were no reasons to believe that irradiation was absent.
It remains to consider other, non-technical hypothesis. Had not the ova a higher radioresistance than spermia with respect to mutability, or X chromosome of the ova than autosomes? This question can be studied by means of comparing the respective frequencies of arising of mutations.
Are not ova with mutations in X chromosome discarded from the pool of all the ova by means of any special mechanism? Is it not known that the chromosome bridges arising from crossing-over inside a paracentric inversion in females heterozygous for such an inversion go to polocytes in meiosis?
The store of verifiable ideas is rapidly exhausted, one has to have approaches to their study, and one can but leave this matter to other researchers.
Briefly, the results of the present work can be summarized as follows. In D. melanogaster, treatment of parental individuals with mutagens affects the mean sex ratio in their immediate progeny very weakly and irregularly, so that as the dose increases, no directed shift in sex ratio is observed. From the sex ratio it is not possible to get an idea of the dose of mutagen and of the number of DLM; therefore, it is quite impossible to estimate the number of DLM in the genome by the sex ratio. In this connection, some earlier works of the author in which it is necessary to correct the most important formulae and values associated with estimation of the number of DLM in the genome have been revised. The present work will serve to indicate the reader necessary corrections.
The work confirms the well-known gnoseological principle, which is, however, often violated. In cognition, logic alone is quite insufficient. Speculations, even the most obvious ones, require checking with facts, because the reality turns out to be richer than any imagination. Empirical generalizations can destroy most perfect theories.
Acknowledgments: The author is sincerely grateful to Dr. V.G. Kolpakov for translation of the paper into English, and to Drs. D.A. Afonnikov and T.M. Mishchenko for their help in preparation of the manuscript for publication.
References: Catcheside, D.G., and D.E. Lea 1945, Journal of Genetics 47:1-9; Fano, U., and M. Demerec 1941, Genetics 26:151; Hadorn, E., 1961, In: Developmental Genetics and Lethal Factors, London: Methuen & Co, New York: John Wiley & Sons; Ivanov, Yu.N., 1998, Dros. Inf. Serv. 81:a) 180-186, b) 186-193, c) 193-197; Ivanov, Yu.N., 1999, Dros. Inf. Serv. 82:87-90; McClintock, B., 1941, Genetics 26:234-282; Taylor, I.T., 1987, In: In the Minds of Men, Toronto: TFE Publishing.